Equilibrium
Law of Chemical Equilibrium and Equilibrium Constants, Henry's Law
Law of mass action : (by guldberg and waage)
A + B → Products
rate ∝ [A] [B]
[A], [B] are the active masses of A and B.
Rate = k[A] [B] , where k = rate constant
Active mass :
Molar concentration = \frac{n}{V_{L}} for both gases and solution. For gases active mass can be expressed in partial pressures also.
8.5 gm of NH3 is present in 500ml vessel
Active mass of \left[NH_{3}\right] = \frac{wt}{m.wt}\times \frac{1000}{V_{ml}}
=\frac{8.5}{17}\times\frac{1000}{500} = 1
rate = k[A] [B] ⇒ k = \frac{rate}{[A][B]}
⇒ rate ∝ k
Equilibrium constant in terms of partial pressure (Kp) :
k_{p} = \frac{P_C^c \ P_D^d}{P_A^a \ P_B^b}
Relation between kp and kc
PV = nRT ⇒ p = \left(\frac{n}{v}\right)RT
P = CRT
k_{p} = \frac{\left(C_{C}RT\right)^{C}.\left(C_{D}RT\right)^{D}}{\left(C_{A}RT\right)^{A}.\left(C_{B}RT\right)^{B}}
k_{p} = k_{c}RT^{\Delta n}
Arrhenius equation with equilibrium constant :
According to Arrhenius k = A.e^{\frac{-E_{a}}{RT}}
A → frequency factor
R → Gas constant
T → Temperature
Ea → Activation energy
\log k = \log A - \frac{E_{a}}{2.303 \ RT}
\log\frac{k_{2}}{k_{1}} = \frac{E_{a}}{2.303 \ R}\left[\frac{1}{T_{1}} - \frac{1}{T_{2}}\right]
\log\frac{k_{2}}{k_{1}} = \frac{\Delta H}{2.303 \ R}\left[\frac{1}{T_{1}} - \frac{1}{T_{2}}\right] Van't Hoff reaction
ΔH = enthalpy of reaction
k1, k2 → equilibrium constant
Case 1 : If ΔH = 0
logK2 − logK1 = 0 : k1 = k2
Case 2 : If ΔH > 0 endo
k2 > k1
Prediction of extent of completion of reaction :
kc > 1 forward reaction is favourable
kc < 1 backward reaction is favourable
A + B \rightleftharpoons C + D
k_{c} = \frac{[C] [D]}{[A][B]}
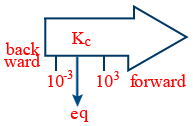
∴ kC = 103 forward favours
kc = 10−3 backward favours
If kc = 1 equilibrium
Reaction quotient (QC):
A + B \rightleftharpoons C + D
Q_{C} = \frac{[C] [D]}{[A][B]} and Q_{P} = \frac{P_{C}\times P_{D}}{P_{A}\times P_{B}}
case I : If QC = kC , reaction is in equilibrium
Case II : If QC < kC forward reaction
Case III : QC > kC back ward reaction
Part1: View the Topic in this Video from 0:11 to 11:26
Part2: View the Topic in this Video from 3:55 to 14:00
Disclaimer: Compete.etutor.co may from time to time provide links to third party Internet sites under their respective fair use policy and it may from time to time provide materials from such third parties on this website. These third party sites and any third party materials are provided for viewers convenience and for non-commercial educational purpose only. Compete does not operate or control in any respect any information, products or services available on these third party sites. Compete.etutor.co makes no representations whatsoever concerning the content of these sites and the fact that compete.etutor.co has provided a link to such sites is NOT an endorsement, authorization, sponsorship, or affiliation by compete.etutor.co with respect to such sites, its services, the products displayed, its owners, or its providers.
1. For a general reaction, \tt aA + bB \rightleftharpoons cC + dD
Rate of forward reaction \propto \left[A\right]^{a} \left[B\right]^{b} = k_{f}\left[A\right]^{a} \left[B\right]^{b}
Rate of backward reaction \propto \left[C\right]^{c} \left[D\right]^{d} = k_{b}\left[C\right]^{c} \left[D\right]^{d}
Where, kf and kb are rate constants.
2. At equilibrium,
Rate of forward reaction = Rate of backward reaction
k_{f}\left[A\right]^{a} \left[B\right]^{b} = k_{b} \left[C\right]^{c} \left[D\right]^{d}
\frac{k_{f}}{k_{b}} = K_{c} = \frac{\left[C\right]^{c} \left[D\right]^{d}}{\left[A\right]^{a} \left[B\right]^{b}}
Where, Kc is called the equilibrium constant.
3. For a gaseous reaction, \tt aA + bB \rightleftharpoons cC + dD
K_{p} = \frac{P_C^c \times P_D^d}{P_A^a \times P_B^b}
4. Relation between Kc and Kp
K_{p} = K_{c}\left[RT\right]^{\Delta n_{g}}
Where, Δng = moles of products − moles of reactants (gaseous only)
5. For a general reaction, \tt aA + bB \rightleftharpoons cC + dD
Which is not at equilibrium,
Q_{c} = \frac{\left[C\right]^{c}\left[D\right]^{d}}{\left[A\right]^{a}\left[B\right]^{b}}

