Atoms
Bohr’s Second Postulate of Quantisation, The Line Spectra of the Hydrogen Atom
- Potential energy of \tt \overline{e} in nth orbit. \tt U_{n} = - \frac{1}{4 \pi \varepsilon_{0}} . \frac{ze^{2}}{r_{n}}
- \tt U_{n} = - 27.2\ \frac{z^{2}}{n^{2}} ev
- When electron jumps from n2 orbit to n1 orbit. hν = En2 − En1
- Frequency \tt v = \frac{2 \pi^{2} me^{4}}{(4 \pi \varepsilon_{0})^{2} n^{3}} \left[\frac{1}{n^{2}_{1}} - \frac{1}{n^{2}_{2}}\right]
- Frequency \tt v = RCZ^{2} \left(\frac{1}{n^{2}_{1}} - \frac{1}{n^{2}_{2}}\right) . \left\{R = \frac{me^{4}}{8 \pi \varepsilon_{0} h^{3}c}\right\}
- The wavelength of emitted radiation. \tt \frac{1}{\lambda} = z^{2} R \left(\frac{1}{n^{2}_{1}} - \frac{1}{n^{2}_{2}}\right)
- If electron jumps from n2 to n1. The number of spectral lines emitted \tt N = \frac{(n_{2}-n_{1}+1)(n_{2}-n_{1})}{2}
- Energy level diagram for hydrogen atom.
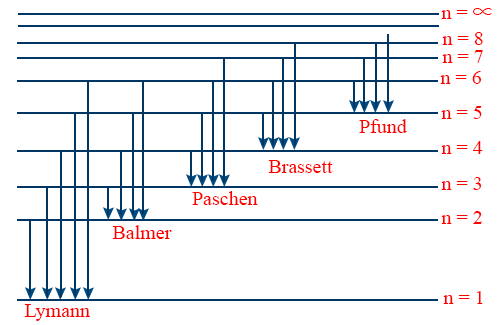
- The wave number of Lyman Series.\tt \overline{V} = \frac{1}{\lambda} = R \left[\frac{1}{1^{2}} - \frac{1}{n^{2}_{2}}\right] (n2 = 2, 3, 4, 5...∞)
- For Lyman Series \tt \frac{1}{\lambda_{max}} = R \left[\frac{1}{1^{2}} - \frac{1}{2^{2}}\right]
- For Lyman Series λmax = 1212 Å
- For Lyman Series \tt \frac{1}{\lambda_{min}}R \left[\frac{1}{1^{2}} - \frac{1}{\infty^{2}}\right]
- For Lyman Series λmin = 912 Å.
- Lyman Series lies in ultraviolet region of electromagnetic spectrum.
- Lyman series is obtained in emission as well as in absorption spectrum.
- Wave number of Balmer series \tt \overline{v} = \frac{1}{\lambda} = R \left[\frac{1}{2^{2}} - \frac{1}{n^{2}_{2}}\right] {n2 = 3,4,5 …}
- For Balmer series \tt \frac{1}{\lambda_{max}} = R \left(\frac{1}{2^{2}} - \frac{1}{3^{2}}\right)
- For Balmer series λmax = 6563 Å
- For Balmer series \tt \frac{1}{\lambda_{min}} = R \left(\frac{1}{2^{2}} - \frac{1}{\propto^{2}}\right)
- For Balmer series λmin = 3636 Å
- Balmer series lies in visible region of Em radiation.
- Balmer series obtained only in Emission spectrum.
- The wave number of paschen series. \tt \overline{V} = \frac{1}{\lambda} = R \left[\frac{1}{3^{2}} - \frac{1}{n^{2}_{2}}\right] (n2 = 4, 5, 6 ….. ∞)
- For paschen series \tt \frac{1}{\lambda_{max}} = R \left[\frac{1}{3^{2}} - \frac{1}{4^{2}}\right]
- For paschen series λmax = 8202 Å
- For Paschen series \tt \frac{1}{\lambda_{min}} = R \left(\frac{1}{3^{2}} - \frac{1}{\infty^{2}}\right)
- Paschen series lies in the infrared region of Em Spectrum.
- Wave number of Bracket series \tt \overline{V}= R \left[\frac{1}{4^{2}} - \frac{1}{n^{2}_{2}}\right] {n2 = 5, 6, ...∞
- For Bracket series \tt \frac{1}{\lambda_{max}} = R \left[\frac{1}{4^{2}} - \frac{1}{5^{2}}\right]
- For Bracket series λmax = 40477 Å
- For Bracket series \tt \frac{1}{\lambda_{min}} = R \left[\frac{1}{4^{2}} - \frac{1}{\infty^{2}}\right]
- For Bracket series λmin = 14572 Å
- Bracket series lies in infrared region of Em. Spectrum.
- Wave number of P fund series \tt \overline{V} R \left[\frac{1}{5^{2}} - \frac{1}{n^{2}_{2}}\right] {n2 = 6, 7 …. 8}
- For P fund series \tt \frac{1}{\lambda_{max}} = R \left[\frac{1}{5^{2}} - \frac{1}{4^{2}}\right]
- For P fund series λmax = 74563 Å
- For P fund series \tt \frac{1}{\lambda_{min}} = R \left[\frac{1}{5^{2}} - \frac{1}{\infty^{2}}\right]
- For P fund series λmin = 22768 Å
- Energy of Hydrogen like atoms. \tt E_{n} = - \frac{13.6}{n^{2}} ev
- E2 = Energy of Second orbit \tt E_{2} = \frac{-13.6}{2^{2}} = - 3.4 ev
- E3 = Energy of Third orbit \tt E_{3} = \frac{-13.6}{3^{2}} = - 1.51 ev
- E4 = Energy of Fourth Orbit \tt E_{4} = \frac{-13.6}{4^{2}} = - 0.85 ev
- Bohr’s theory is applicable only for hydrogen like single electron atom.
- Bohr’s theory could not explain fine features of the hydrogen spectrum.
- Bohr’s theory does not explain why only circular orbits are chosen.
- Excitation energy of an atom is defined as the energy required to jump from the ground state to any one of excited states.
- Ionisation energy is the energy required to knock out an electron completely out of atom.
Bohr's Second Postulate of Quantisation View the Topic in this video From 06:41 To 13:24
Disclaimer: Compete.etutor.co may from time to time provide links to third party Internet sites under their respective fair use policy and it may from time to time provide materials from such third parties on this website. These third party sites and any third party materials are provided for viewers convenience and for non-commercial educational purpose only. Compete does not operate or control in any respect any information, products or services available on these third party sites. Compete.etutor.co makes no representations whatsoever concerning the content of these sites and the fact that compete.etutor.co has provided a link to such sites is NOT an endorsement, authorization, sponsorship, or affiliation by compete.etutor.co with respect to such sites, its services, the products displayed, its owners, or its providers.
1. This is called the Bohr radius, represented by the symbol a0. Thus, a_{0}=\frac{h^{2}\varepsilon_{0}}{\pi\ m e^{2}}
2. If the speed of the electron is much less than the speed of light, the momentum is mvn. Thus, λ = h/mvn. From Eq.(12.24), we have
2π rn = n h/mvn or m vn rn = nh/2π
3.For the Balmer series, the Rydberg constant R is readily identified to be R=\frac{me^{4}}{8\ \varepsilon_{0}^{2}\ h^{3}c}

