States of Matter: Gases and Liquids
Gas Laws and Ideal Gas Equation and Kinetic Molecular Theory of Gases
Gas Laws:
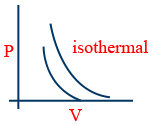
\tt V\propto\frac{1}{P} (Boyle's Law)
PV = constant
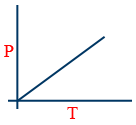
Charles' Law:
V ∝ T (or) \tt \frac{V_{1}}{V_{2}}=\frac{T_{1}}{T_{2}}
Gay-Lussac Law:
\tt \frac{P_{1}}{T_{1}}=\frac{P_{2}}{T_{2}}
Avagadro's Law:
V ∝ n
Ideal Gas Equation:
\tt V \propto \frac{1}{P}
V ∝ T
V ∝ n
\tt \therefore V \propto \frac{nT}{P}
PV ∝ nT
PV = nRT
Graham Law of Diffusion of Gases:
\tt r\propto\frac{1}{\sqrt{d}}\propto\frac{1}{\sqrt{M.wt}}\propto\frac{1}{\sqrt{V.D}} (Constant T and P)
\tt \frac{r_{1}}{r_{2}}=\frac{\sqrt{d_{2}}}{\sqrt{d_{1}}}=\sqrt{\frac{M_{2}}{M_{1}}}=\sqrt{\frac{V.D_{2}}{V.D_{1}}}=\frac{V_{1}t_{2}}{V_{2}t_{1}}
\tt \frac{r_{H_{2}}}{r_{CH_{4}}}=\frac{\sqrt{M_{CH_{4}}}}{\sqrt{M_{H_2}}}=\sqrt{\frac{16}{2}}=2\sqrt{2}
Dalton's Law of Partial Pressure:
Ptotal = P1 + P2 + P3 + ....
P.P = M.F × T.P = Xi × T.P [Xi = mole fraction]
\tt Xi=\frac{P.P}{T.P}
Ptotal = PA + PB + PC = (n1 + n2 + n3) \tt \frac{RT}{V}
Kinetic Gas Equation:
Urms = \tt \sqrt{\frac{u_1^2+u_2^2+u_3^2...+u_n^2}{n}}
K.E equation = \tt PV=\frac{1}{3}MC^2
\tt K.E=\frac{3}{2}RT
\tt K.E=\frac{3}{2}kT (PV=RT)
Part1: View the Topic in this Video from 3:47 to 54:04
Part2: View the Topic in this Video from 1:43 to 53:00
Part3: View the Topic in this Video from 0:56 to 1:00:00
Disclaimer: Compete.etutor.co may from time to time provide links to third party Internet sites under their respective fair use policy and it may from time to time provide materials from such third parties on this website. These third party sites and any third party materials are provided for viewers convenience and for non-commercial educational purpose only. Compete does not operate or control in any respect any information, products or services available on these third party sites. Compete.etutor.co makes no representations whatsoever concerning the content of these sites and the fact that compete.etutor.co has provided a link to such sites is NOT an endorsement, authorization, sponsorship, or affiliation by compete.etutor.co with respect to such sites, its services, the products displayed, its owners, or its providers.
1. Boyle's law
PV = K or \tt P_{1}V_{1} = P_{2}V_{2} = K (For two or more gases)
2. Boyle's law can also be expressed as \tt \left(\frac{dP}{dV}\right)_{T} = \frac{-k}{V^{2}} \ or \ \frac{dV}{V} = \frac{-dP}{P}
3. \tt K = \frac{V}{T} \ or \ \frac{V_{1}}{T_{1}} = \frac{V_{2}}{T_{2}} = K \left(For \ two \ or \ more \ gases\right)
4. Charle's law can be represented as \tt \left(\frac{dV}{dt}\right)_{P} = K
5. \tt K = \frac{P}{T} \ or \ \frac{P_{1}}{T_{1}} = \frac{P_{2}}{T_{2}} = K \left(For \ two \ or \ more \ gases\right)
6. Avogadro's Law: \tt \frac{V_{1}}{n_{1}} = \frac{V_{2}}{n_{2}} = ............ = K
7. PV = nRT, This is called ideal gas equation.
8. If a number of gases having volume V1, V2, V3 ...... at pressure P1, P2, P3 ....... are mixed together in container of volume V, then,
\tt P_{Total} = \frac{P_{1}V_{1} + P_{2}V_{2} + P_{3}V_{3}......}{V}
or = \tt \left(n_{1} + n_{2} + n_{3}.......\right)\frac{RT}{V} \ \left(\because PV = nRT\right)
or = \tt n\frac{RT}{V} \ \left(\because n = n_{1} + n_{2} + n_{3}.......\right)
9. \tt \frac{r_{1}}{r_{2}} = \sqrt{\frac{d_{2}}{d_{1}}} = \sqrt{\frac{d_{2} \times 2}{d_{1} \times 2}} = \sqrt{\frac{M_{2}}{M_{1}}}
Where, M1 and M2 are the molecular weights of the two gases.
10. For gases \tt \frac{V_{1}}{V_{2}} = {\frac{n_{1}}{n_{2}}}
\tt {\frac{n_{1}}{n_{2}}}\times{\frac{t_{2}}{t_{1}}}= \sqrt{\frac{M_{2}}{M_{1}}}
11. When equal volume of the two gases diffuse, i.e. V1 = V2 then, \tt {\frac{r_{1}}{r_{2}}} = {\frac{t_{2}}{t_{1}}}= \sqrt{\frac{d_{2}}{d_{1}}}
12. When volumes of the two gases diffuse in the same time, i.e. t1 = t2 then, \tt {\frac{r_{1}}{r_{2}}} = {\frac{V_{1}}{V_{2}}}= \sqrt{\frac{d_{2}}{d_{1}}}
13. Kinetic gas equation : On the basis of above postulates, the following gas equation was derived,
\tt PV = \frac{1}{3}mnu_{rms}^2
where, P = pressure exerted by the gas
V = Volume of the gas
m = average mass of a molecule
n = number of molecules
urms = root mean square (RMS) velocity of the gas.

