Moving charges and magnetism
Motion in Combined Electric and Magnetic Field
When a moving charge is acted upon by electric and magnetic fields, the charge experiences a force called Lorentz force, which is a vector sum of forces due to electric and magnetic fields.
When the electric field, the magnetic field, and the motion of charge are mutually perpendicular to each other( as shown in the fig )then they are called as crossed fields and forces due to electric and magnetic fields will act in the opposite directions so the Lorentz force F will be
F = qE \hat{i} + \left(q V \hat{i} \times B \hat{k} \right) = q E \hat{j} - q VB \hat{i} = q (E - VB) \hat{j}
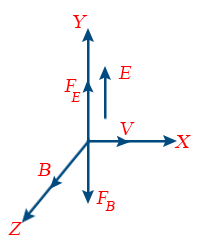
When the strength of electric and magnetic fields are varied to get the forces due to electric and magnetic field to be equal then the charge can move in the field without any deflection
q E = qv B
V = E/B.
View the Topic in this video From 00:55 To 57:00
Disclaimer: Compete.etutor.co may from time to time provide links to third party Internet sites under their respective fair use policy and it may from time to time provide materials from such third parties on this website. These third party sites and any third party materials are provided for viewers convenience and for non-commercial educational purpose only. Compete does not operate or control in any respect any information, products or services available on these third party sites. Compete.etutor.co makes no representations whatsoever concerning the content of these sites and the fact that compete.etutor.co has provided a link to such sites is NOT an endorsement, authorization, sponsorship, or affiliation by compete.etutor.co with respect to such sites, its services, the products displayed, its owners, or its providers.
Force on a Charged particle in Combined uniform Electric and Magnetic fields
\overrightarrow{F} = \overrightarrow{F}_{E} + \overrightarrow{F}_{M} = q\overrightarrow{E} + q(\overrightarrow{v} \times \overrightarrow{B}) = q (\overrightarrow{E} + \overrightarrow{v} \times \overrightarrow{B})

